Author Archives: admin

Crazy Transformation Examples

The parent graph (a.k.a. library function) graphing examples in this video are more towards the psycho-hard end of the spectrum, bringing up to four transformations at once to each of the library functions (square roots, parabolas, cubics, etc). Up shift and left shift and vertical stretch and reflection you say? Hold on to your hat!

Piecewise Functions

They should be called the Frankenstein Functions, because they're monstrosities cobbled together from pieces of other library functions: lines, parabolas, square roots, cubics, cube roots, and absolute value. Like the actual Frankenstein, piecewise functions are more vulnerable if you break them into their constituent parts.

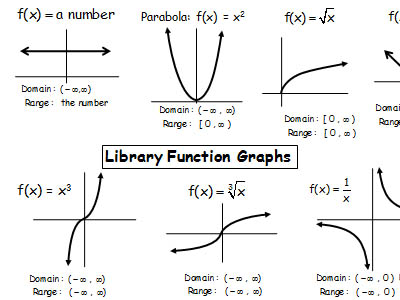
Intro to "Library Function" Graphs (a.k.a. "Parent Functions"): square roots, parabolas, cubics, etc

(click for printable PDF LIBRARY FUNCTIONS CHART)

Intro to Graphing Transformations

Graphing crazy library/parent functions with stretch, flips, and vertical and horizontal shifts is pretty confusing for most students. So, in this video we'll start by applying transformations to a function everyone is already comfortable with -- parabolas (y=x²) -- to get the hang of it before moving onto other weird shapes. By the end of this video you'll be able to move a parabola up, down, left, right, inverted & stretched!

Finding X and Y Intercepts

Turns out finding intercepts of a function is done the same way whether you're working with lines or high-power polynomial nightmares. In this chapter, naturally, we'll be working with the easier stuff, though we'll keep revisiting the topic (as will your teacher) for years to come.

Inverse Functions

Believe it or not, you've been using inverse functions since you solved your first algebra equation. (Multiplication is the inverse of division, addition is the opposite of subtraction.) In this long video we'll get into a step-by-step process to find inverse functions, graph inverse functions, and anticipate and assess their function-hood.

Composite Functions

Like the similarly-named sketches which assist the police in tracking down suspects, these combinations of various functions will "arrest" you in a fit of confusion as you ponder the domain of g(f(x)). (Pun very much intended. You're welcome!)

Domain & Range

The domain of a function is all it's x-values, usually an interval. Range is the set of y-values. In this video I cover how to find domain and range using the two big rules you never want to break: dividing by zero, and square rooting a negative. Don't do it!

Graphing Functions By Plugging In

There are lots of fancy ways to graph functions, and we'll get into that in other videos. But the fall-back method you'll be happy to have in your back pocket is "plugging s*@#! in. (That was "stuff", what did you think I meant?)"

Intro to Functions

In this video we'll introduce lots of concepts of functions, such as: what "f(x)" means, function notation, the vertical line test, "one-to-one" functions, how to plug numbers into a function, and even those silly problems where they give you a bunch of points and ask if they're a function.

Equations of Parallel & Perpendicular Lines (free)

The SAT, in particular, emphasizes being able to find the equation of a line perpendicular to another line. [hint: "negative reciprocal" is gonna help] Later math and science classes use this property too, so it's not a complete waste of time.

Point-Slope Form:
y-y₁=m(x-x₁)

I saved this for last because I want it to leave a lasting impression. From here on out, no matter what direction your academic career takes, this is the form of a line that will serve you the best in calculus, econ, business, you name it. Slope-intercept, step aside.

Standard Form of a Line: Ax+By=C

Not sure what's so "standard" about it. It has almost no meaning, and there's no situation in which one of the other two forms wouldn't be better! Yet they make you learn it, perhaps as some sort of throw-back to algebra methodologies of days gone by.

Slope-Intercept Form of a Line: y=mx+b

This is the line equation that everyone learns first, and therefore it's the first one that all my tutoring students try to use whenever lines are involved. Though that choice is yours, I will advocate for point-slope in the next video, because it really is better.

Horizontal & Vertical Lines

This video gets into the nitty gritty of horizontal and vertical lines. Why is there only one letter in them? How do you find the equation of a vertical line through a point? Why is the slope of a horizontal line zero, while vertical is undefined?

How to Find the Slope of a Line

Calculating slope, graphing slope. This video also gets into the slope special cases a bit, such as the slopes of vertical, horizontal, and perpendicular lines.

How To Graph Lines (a.k.a. Linear Functions)

In this video we introduce the main standard equations of lines, and talk about how to graph them. The main takeaway will be: when in doubt, either solve for the x- and y-intercepts, or plug in a few x's to find a few points on the line, then connect the dots.